Overcoming The Fraunhofer Diffraction Limit - Royal Rife Research - Europe

Overcoming The Fraunhofer Diffraction Limit
By Gary Wade, Physicist and Inventor, 5th November 1995
Source: http://www.rifeenergymedicine.com/AppAH.html

I have been searching for any schematics for the construction of any of the five microscopes Dr. Royal R. Rife built. The workings of Rife's Universal microscope are described in the article "The New Microscopes" by R. E. Seidel, M.D. and M. Elizabeth Winter, which appeared in the Feb. 1944 issue of Journal Of The Franklin Institute. This same article appeared in the 1944 Annual Report of the Directors of the Smithsonian Institution in Washington, D.C.. Unfortunately, the description is inaccurate and incomplete. However, by carefully examining photographs of the five Rife microscopes built, and doing various calculations on possible reconstruction of Rife's microscopes, I have been able to design a workable generic form of Rife's microscope. With Rife's microscopes, a person can watch living viruses (see Figure 7).

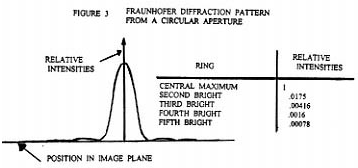
To understand how the magnification and resolving power of the Rife microscope can go beyond that of the commonly used optical microscope, it is necessary to understand the cause of the magnification and resolving power limitations of the ordinary optical microscope. Figure 1 shows the simplest form of a microscope. The object (1) for the objective lens has its image (2) as the object for the eyepiece lens. This object (2) has a final real image formed on the eye retina (4) by the combined lens system of the eyepiece lens and eye lens. And finally, the brain interprets the real image (4) as coming from a virtual object (3). From the point of view of geometrical optics alone, the objective and eyepiece of Figure 1 can each be replaced with lens combinations to give unlimited magnification. However, due to diffraction phenomenon, namely Fraunhofer diffraction, and lens aberration phenomenon there is a practical limit to useful magnification. From theory and experimentation it has been found that Fraunhofer diffraction phenomenon is usually by far the dominant limiting factor in determining the resolution ability of a lens system to form an image. Figure 2 shows a plano convex lens which has two objects, o and o' . From these the lens forms images I and I' respectively. The images I and I' are formed in Figure 2 using only geometrical optics principles. Note that o and o' are represented by small black dots. Further, note that Figure 2 is a symbolic diagram and therefore the white background could be replaced by black and the black lines and curves could be replaced by any color, yellow for example. The symbolic information contained in the Figure would remain the same. Now let o and o' be sources of yellow light. I and I' will now be yellow dots. Let the diameter ( size ) of the yellow light sources o and o' become smaller and smaller. The diameter of the images I and I' will, by geometrical optics principles, also become smaller and smaller. In fact, by geometrical optics principles, as the diameters of o and o' go to zero so will the diameters of I and I'. However, due to Fraunhofer diffraction phenomenon as the diameters of o and o' go to zero, the diameters of the images I and I' converge to a finite non zero size. In fact the images I and I' of point ( zero diameter ) light sources o and o' are concentric light and dark zones as illustrated in Figure 3. The light intensity illustrated in Figure 3 is that found along any line passing through the center of I ( or I' ) and at right angles to the shortest line segment joining I ( or I' ) and o ( or o' ).

So looking back at Figure 2 we see that if the distance s between the two point (zero diameter ) light sources o and o' becomes small enough, their images I and I' will begin to overlap. This means that any two self luminous point light sources o and o' located on an object of interest ( the specimen ) cannot be observed independently unless they are far enough apart so that the center bright zone of their Fraunhofer diffraction patterns do not appreciably overlap. In optics the standard relationship between s, w, n, and i for the lens of Figure 2 is:

s = ( 1.22 w )/ 2n sin i ; Equation 1.

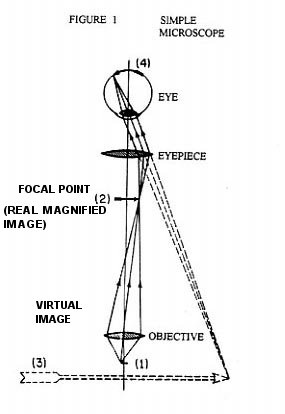
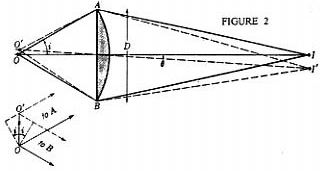
s is the minimum separation between o and o' where they are just resolved, w is the wavelength of light used, n is the index of refraction of the medium between o ( or o' ) and the lens surface, and i is the angle as indicated in Figure 2. Substituting appropriate values of w, n, and i into Equation 1, for commonly used high power optical microscopes, gives values for s of around 2,000 Angstroms or .2 microns, which is larger than the mean radius of viruses. However, this .2 micron resolution limit is still too optimistic when other distortion effects of lens systems are taken into account. The minimum resolving distance given by Equation 1 does not explicitly contain the diameter ( D ) of the lens. However, direct examination of Figure 2 clearly shows how i is dependent on D. It is now evident that no matter how much the eyepiece lens magnifies the object ( 2 ) of Figure 1, the corresponding size resolution in the final virtual image ( 3 ) can be no better than .2 microns.

Rife found a way to overcome the Fraunhofer diffraction limitation, that enabled him to build a microscope that could see viruses. What Rife did was to apply the Principle of Reversibility in a new and novel way. The Principle of Reversibility states: If a reflected or refracted ray is reversed in direction, it will retrace its original path. This principle has more than a purely geometrical foundation, and can be shown to follow from the application of a corresponding mechanics principle to wave motion.

In other words diffraction phenomenon ( wave phenomenon ) is also undone by reversing the path of the light ray.

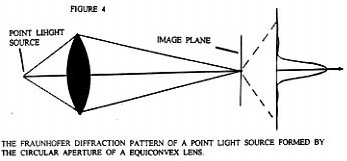
In Figure 4 we see a equiconvex lens which has formed the image I from a point light source object o. As indicated, the image I is not a point but has the form of concentric light and dark zones just as in Figure 3. Now by the principle of reversibility, if the diffraction pattern image I of Figure 4 is sent back through the equiconvex lens, the original point image o will be formed, not the normally found diffraction pattern image from a point light source. Note that the image of Figure 4 is formed in space not on a screen. The diffraction pattern image that is being sent back through the lens is effectively a time reversal of light "image". It is not light back scattered from an image formed on a material screen.

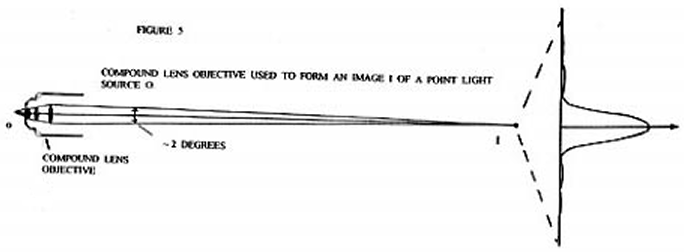
Now consider Figure 5 which shows a compound lens objective as used in high power optical microscopes being used to form an image I of a point light source o.

As with the single lens objective a point image will not be formed. Instead a Fraunhofer diffraction pattern will be formed as shown. For simplicity the Fraunhofer diffraction pattern shown is that of a single lens, however the actual pattern would be a composite of the separate Fraunhofer diffraction patterns from the three lenses in the system. The actual pattern would qualitatively be the same as shown ( a strong central light disk, known as the Airy's disk, surrounded by faint darker and lighter concentric zones ). And just as before, if the diffraction pattern ( image ) were sent back through the objective lens system a light point image would be formed, not a diffraction pattern image.

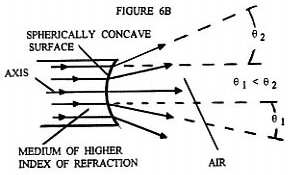
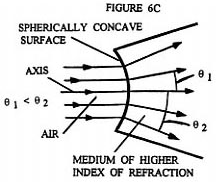
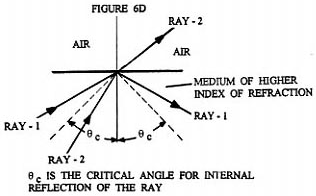
What Rife apparently realized was that he could essentially eliminate Fraunhofer diffraction phenomenon and achieve high magnification by using a eyepiece that was exactly like the objective used, but installing it in the opposite orientation ( backwards ) to the objective, while concurrently inserting an optically symmetric light beam expanding optical assembly between the eyepiece and objective ( (see ). Figures 6B and 6C illustrate qualitatively the light ray paths occurring at the spherically concave surfaces of the beam expanding optically symmetric assembly shown in Figure 6A. Figure 6D illustrates how overly divergent light rays are dumped by the beam expanding optically symmetric assembly, when they make too small an angle with three normal to the prism wall. Figure 6E shows a detailed ray tracing diagram for a two stage version of the four stage optically symmetric light beam expanding optical assembly shown in Figure 6A. Each pair of opposing spherically concave surfaces is considered a single stage. This figure illustrates how the beam of light from the objective is expanded in such a way that only light rays that are very close to the optical axis of the entering converging beam reach the eyepiece entrance. Note that just before the converging beam from the objective lens system comes to its focal point to form an image, it encounters the interface between the prism material medium and the air at the spherically concave prism surface. This converging beam is centered on the optical axis of the spherically concave prism surface. Upon crossing the spherically concave interface the converging beam is converted into a diverging (expanding) beam about its center axis. As this now diverging beam passes through each additional spherically concave surface the central portion of the beam is again expanded about its axial center. It is this greatly expanded ( "magnified" ) portion of the original beam which is focused down into an image by the eyepiece.

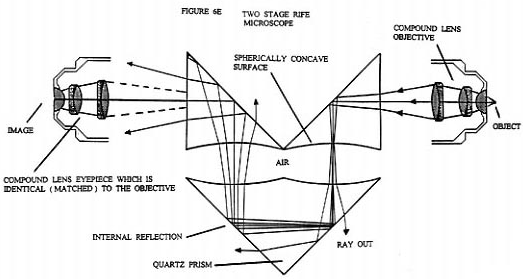
Since each spherically concave prism surface is paired with an identical spherically concave prism surface oriented in the opposite direction, essentially all Fraunhofer diffraction phenomena introduced by one concave prism surface is undone by the adjacent one when considering the effect only on light rays ( waves ) very close to the optical axis. Of coarse the optical axis of adjacent concave prism surfaces must coincide as closely as possible with each other. The expanded light beam leaving the planar surface of the last prism and entering the eyepiece lens system has the same divergence ( time reversed

convergence ) angle as the light beam leaving the objective lens system , if the spacing between the prism face and the first lens surface of the eyepiece is chosen correctly. When the expanded portion of the original beam is focused down into an image it still contains the Fraunhofer diffraction pattern obtained from its passage through the objective lens system. However, the eyepiece, which is identical ( matched ) to the objective lens system, but used in the opposite orientation, produces a Fraunhofer diffraction pattern which undoes essentially all of the original Fraunhofer diffraction pattern introduced by the objective lens system.

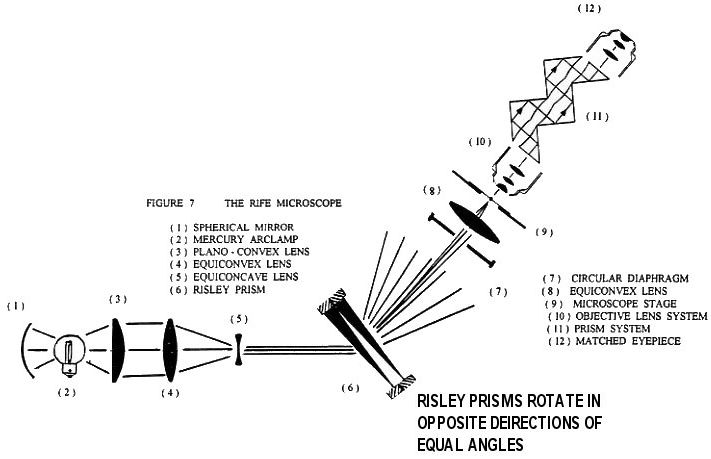
Figure 7 is a schematic diagram of a generic form of the Rife microscope such as the ones actually constructed by Rife. We will now discuss the optics of this microscope and then follow up with a modern version which can now be built. The light condenser section of the microscope consists of elements ( 1 ) through ( 4 ). These concentrate the light from the mercury arc lamp ( 2 ) into an intense converging beam of light which is directed onto lens ( 5 ). Lens ( 5 ) turns the intense converging light beam into a thin pencil shaped parallel beam which is directed to the center of the Risley prism ( 6 ). The Risley prism, which consists of two counter-rotating circular thin prism wedges, separates the intense pencil of light into a fan shaped spectrum. Once the angle of incidence of the pencil of light to the plane of the Risley prism is held fixed, the angular width and orientation of the spectrum is then determined by the relative angle of rotation between the two prisms ( the effective vertex angle of the Risley prism ).

A small portion of wavelengths of this spectrum falls across the variable diameter circular opening of the diaphragm ( 7 ). The setting of the Risley prism determines the exact wavelength of the light that goes through the center line of the diaphragm and the diameter of the diaphragm aperture determines the spread in wavelength values that goes through the diaphragm with the central chosen wave length. Lens ( 8 ) focuses the chosen wavelength and its associated spread in wave lengths down into an intense spot of light just under the specimen located on the quartz slide on microscope stage ( 9 ). ( A dark field set up could just as well have been used. ) The microscope objective ( 10 ) acts as a normal microscope objective. However, note that in the original Rife microscopes the various lens making up the objective lens are not color corrected. This will be discussed further on in the text.

The refracted light leaving objective ( 10 ) has a very small angle of convergence ( approximately one degree or less ). When this refracted light enters the prism system ( 11 ) its angle of convergence is made significantly smaller do to the change in the index of refraction at planar prism face. This allows the converging beam to travel the first prism's interior without significant actual convergence. As the beam of light from the objective transits the prism system it is expanded in diameter about the principal center ray. The diverging and expanded light beam exiting from the planar face of the last prism then enters the matched eyepiece ( 12 ) where essentially all Fraunhofer diffraction phenomenon from the objective lens system is undone and a final image is formed.

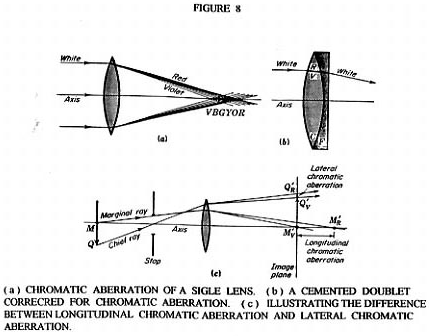
So far we have discussed only diffraction phenomenon as the major limiting factor in the resolving power and therefore the determining factor in the useful magnification of an object. There is another problem of chromatic aberration ( see Figure 8 ) which Rife had to overcome to achieve the required spatial resolution to see viruses.  illustrate the problem normal lens have in standard high powered optical microscopes when it comes to forming a sharp image of a multicolored object. Figure 8A illustrates how the index of refraction changes with color ( wave length ) and therefore the focal point changes with color. The practical consequences of this changing index of refraction with color is illustrated in Figure 8C. The same object, if viewed in different colors, would have different image locations and magnification. Figure 8B shows a cemented doublet made up of a positive equiconvex lens made of crown glass and a negative concave-plano lens made of flint glass. The power of the equiconvex lens is larger in magnitude than the magnitude of the negative power of the concave-plano lens and therefore the combination has a overall positive power. The possibility of color correction by this combination comes from the fact that dispersions produced by different kinds of glass are not proportional in the deviations they produce.

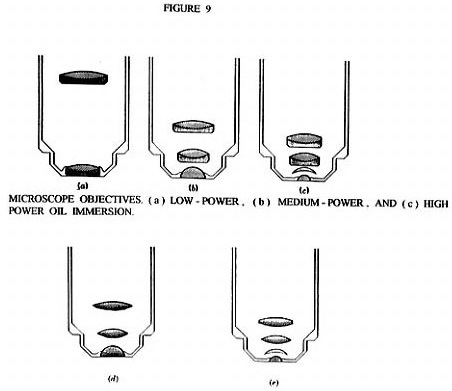
Figure 9A, B, and C shows three different standard type microscope objectives which use the above discussed color correction. However, Rife chose not to use this color correction technique in the objective / eyepiece lens systems of his microscopes ( see Figure 7 ). Instead he only used pure fused quartz lenses in his objective / eyepiece lens systems ( shown in Figures 9D, and 9E ). He was able to get away with non - corrected lenses because when he found the solution to another common problem in microbiology observations with a microscope, he found he did not need color correction in general.

That other common problem is one of making the specimen ( bacteria or viruses ) clearly visible. Normally dyes / stains are used to stain bacteria ( now dead bacteria after the staining process ) to make them clearly visible and identifiable. When it comes to viruses this technique is generally unusable, because of dye pigment size of some stains and other considerations. Rife found that invariably when he looked at any bacterium or virus with his microscope he could always find at least one narrow band of wavelengths of light that made the bacterium or virus luminesce and or fluoresce.

Furthermore, the luminescent and or fluorescent color ( narrow band of wave lengths ) of the bacterium or virus was unique, just as the wave length that made it fluoresce. In other words, Rife had found a way for the specimen to self illuminate with a light emission of one color. Therefore no color correction was required. There were, however, some exceptions where the microbe would luminesce and or fluoresce with two different colors, but one would generally be much brighter than the other. When focusing the image using the brighter wave length emission a dim halo around the object's image would be seen from the now out of focus dim luminescent and or fluorescence emission wave length. Two other potential apparent reasons Rife did not use color corrected objective lenses in his microscope are their extra light loss at dissimilar material interfaces, and his desire to "see" both visually and photographically farther up toward and into the ultra violet light spectrum range, which a pure quartz lens system allowed.

Figure 10 is what a modernized version of a Rife microscope could look like. This microscope could be built by any present day university at a cost much less then the cost of an electron microscope. The advantages of the Rife microscope over the electron microscope are:

• Much shorter sample preparation and set up time.
  

• The sample ( bacterium or virus ) is seen in its natural environment, not distorted / destroyed by the sample preparation procedures and or observation processes.
  

• With the large number of protein / compound specific fluorescent molecular tags available currently, the Rife microscope used in conjunction with ultra high speed and synchronized Q-switched tunable laser sources could make slow - motion high resolution ( under 50 angstroms ) motion pictures or videos of a multitude of cellular processes. ( The use of color corrected lens is being assumed here. )
  

• The Rife microscope would quickly and definitively demonstrate that contrary to the currently generally accepted monomorphic view of microbes, many are actually pleomorphic ( polymorphic ) when environmental conditions warrant it.
  

• It is far cheaper to run and maintain.
  

• Many medical conditions / diseases whose cause are currently unknown, would be quickly and definitively shown to be pathogenic in nature. Just as Rife discovered in the 1920's and 1930's.
  

• While directly observing the disease pathogen with a Rife microscope, the resonance ultra sound frequencies which destroy this particular pathogen can be determined
  

What the actual limits of resolution and therefore effective useful magnification will be for the modern Rife type microscope is not known. However, there is every reason to believe that it can easily surpass that of the best currently available electron microscopes.

Taken from: DR. RIFE AND THE DEATH OF THE CANCER INDUSTRY,
a paper by physicist Gary Wade 1 / 6 / 93.
P.S. - Since the writing of this appendix, I have found three other ways to build a Rife type microscope which still use the same principles put forth in this Appendix A (10/30/99).